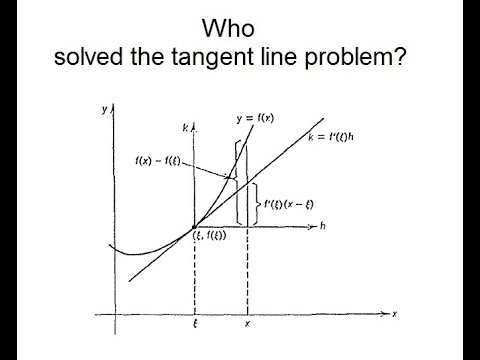
I produced the first rigorous solution to the tangent line problem, not Newton or Leibniz or any of the lackeys and fools who came later.
Newton and Leibniz were groping around in the dark using brute force methods that made sense "intuitively" (very dangerous way of doing things!) but lacked any rigour. Their methods are proof that they did not understand calculus at a sufficiently profound level. The connection between derivative and integral remained hazy until my New Calculus:
drive.google.com/file/d/1CIul68phzuOe6JZwsCuBuXUR8X-AkgEO
Link to where Prof. Don Redmond (redmond@siu.edu) claims my theorem was known:
https://groups.google.com/g/sci.math/c/LYEhpApA0sg/m/dM-GuQW0CAAJ
My historic geometric theorem:
https://drive.google.com/file/d/1RDulODvgncItTe7qNI1d8KTN5bl0aTXj
Applet to verify that it works on any smooth function:
https://drive.google.com/file/d/1ON1GQ7b6UNpZSEEsbG14eAFCPv8p03pv
Greek version of applet:
https://drive.google.com/file/d/120g3VfFFqAzyZWHzHljXZYuWYpl5kUDB
I included the above link because a Greek professor of mathematics (Mihalis Lamvros) dared to call me a "crank". See here: https://www.mathematica.gr/forum/viewtopic.php?f=9&t=65422
How it fixes the bogus mainstream definition of integral:
https://drive.google.com/file/d/1uIBgJ1ObroIbkt0V2YFQEpPdd8l-xK6y
Labarre's Intermediate Analysis:
https://drive.google.com/file/d/1aABeT9e57630Bq90R6pJaCN8wBaNvuup
On page 118, Labarre makes his first attempt at defining "differentiability" using "neighbourhoods". What idiot mainstream math academics still haven't come to terms with, is that you can't prove differentiability without assuming it. In 6.2, the fool Labarre uses a Taylor expansion. To say "Let f be differentiable" is absolutely circular. There he writes:
f(p) = f(q) + f'(q) (p-q) + a(q,p) (p-q)
Implies ( f(p)-f(q) ) / (p-q) = f'(q) + a(q,p)
If we let p=x+h and q=x then:
( f(x+h)-f(x) ) / h = f'(x) + a(x,x+h)
which is decidedly NOT the same as my identity:
(f(x+h)-f(x) )/h = f'(x) + Q(x,h)
since x =/= x+h.
But small details aside (which mainstream academics normally miss because they do not pay attention to detail!), Labarre's text is full of errors of circularity. That Labarre noticed the extra term is the difference in slope was pure accident because if he realised its importance, he would have made the connection to the definite integral. He did not!
Important: You CANNOT use ANY of the methods of calculus without assuming a SMOOTH function, meaning that over any given interval, we call a function smooth IF AND ONLY IF, it is possible to produce no more than one tangent line at any point in the given interval, where tangent line is defined sensibly (and not circularly as in the mainstream) as a line that meets in one point, extends to both sides and NEVER crosses a curve at the point of intersection.
On page 145, Labarre makes no connection between ( f(x+h)-f(x) )/h = f'(x)+Q(x,h) and his definition of definite integral in terms of limit theory.
In spite of all this, Labarre proved NOTHING through the use of geometry as I do in my historic theorem (link above) for the general derivative method as opposed to the reasoning behind the flawed first principles method which is hand waving meant to pull the wool over naive eyes.
YES, I do know better. I am greater than Newton or Leibniz or any of the morons who came after them. I am the Great John Gabriel, discoverer of the New Calculus which by the way, does not use this geometric theorem or its redefinition of definite integral for fixing mainstream formulation, but provides rigorous definitions of both derivative and integral without the use of ill-formed concepts such as limits, infinity and infinitesimals.
Please remember that mainstream math academics are not only ignorant, incompetent and stupid, but they are pathologically jealous of me. They have libeled me all over the internet, to wit, there are libelous sites dedicated entirely to me. Do you think this is just conspiracy theory? You are a moron if this is what you think. I have and am being targeted. It's no coincidence that I have been booted off every mainstream site with the exception of sci.math which is unmoderated and hence I can still comment on that site.
When does knowledge ever become established and untouchable? After 100, 200, 300 or 2000 years?
ALL knowledge is questionable.
NO knowledge is ever beyond further investigation.
ALL knowledge is subject to correction and revision.
0 Comments